Inverse problems in physical and biological sciences and biomedical imaging

Near infrared (NIR) light provides characterization and diagnostics of biological tissue and cells which is noninvasive, safe, of low cost, and easy to use. NIR light can penetrate deep (~5cm) into tissue. By probing the tissue with NIR light at multiple wavelengths, the concentration of the chromophores (oxy- and deoxy-hemoglobins, water and lipid) of tissue can be reconstructed from light attenuation within the tissue because the spectra of NIR light absorption by different chromophores differ significantly. The physiological and functional information of tissue can then be visualized in a three-dimensional (3D) image. The structural change caused by carcinogenesis results in the change of scattering property of tissue and can also be probed by light. With the current development in molecular beacons and other advanced fluorescence agents, light can detect tissue changes at the molecular level. However, strong scattering of light by tissue obscures direct images and inverse reconstruction is required to form images.

Inverse problem is ubiquitous in physical and biological sciences where unobservables are deduced from observable quantities. Moreover, inverse problem is inevitable in imaging a heterogeneous medium. In biomedical optical imaging where breast, prostate, or brain and etc is being examined, challenges exist in both modeling accurately light propagation in biological tissue and in dealing with the ill-posedness of the inverse problem.

My research on optical imaging consists of developing a more accurate forward model than the commonly used diffusion approximation and advanced reconstruction methods. The cumulant solution to radiative transfer improved upon the diffusion approximation in optical imaging [1, 2, 3]. In the area of inverse reconstruction, several fast three-dimensional image reconstruction algorithms have been developed based on symmetry considerations and Fourier techniques [4, 5, 6], and recently a new scheme for optical imaging using independent component analysis (OPTICA) has been introduced [7, 8, 9, 10].

OPTICA interprets optical imaging as a source separation problem and uses independent component analysis to sort out the inhomogeneities within the turbid medium. OPTICA is fast and has several salient features compared to conventional optical imaging methods. In particular, a prior assumption about how light propagates in the medium is not needed to find out the inhomogeneities. A model for light propagation is only required in a Green’s function analysis of the retrieved independent components to locate and characterize the inhomogeneities. Unlike the conventional optical imaging methods, OPTICA doesn’t require an accurate modeling of light migration across short distances. Both simulations [8] and experiments [7, 9, 10] have shown OPTICA works best for small absorption, scattering, or fluorescence inhomogeneities (~ 1 - 10mm in size) and resolves the position and optical properties of localized inhomogeneities to a high degree of accuracy. This approach works extremely well for fluorescence inhomogeneities. OPTICA should become an ideal imaging approach for molecular imaging. The potential of OPTICA for molecular imaging and multiple wavelength spectral imaging are currently being investigated. OPTICA should also be very useful in near-infrared optical imaging and spectroscopy in the study of human brain activation and will not suffer from the partial volume problem. A companion approach “time reversal optical tomography” has recently been introduced [11].

(a)

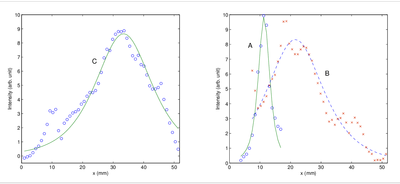(b)

Figure 1: (a) Independent intensity distribution on the detector plane (z = 33 mm) obtained by OPTICA for the tumor, C (left pane), and the glandular structures A and B (right pane). (b) Corresponding bottom panes show the Green's function fits (solid lines) to the horizontal spatial profile (denoted by circles and crosses) through the center of the intensity distributions along the dashed lines.

Figure 2: Left pane: The cross section image of the tumor at the z = 18.2 mm plane formed by back-projection. Right pane: Spatial profiles of the cross section image along the x and y directions shown by the white dashed lines. The FWHM of the cancer site is 10.3 mm and 7.4 mm along the x and y directions, respectively.

Modulated Imaging

Recent advances in light modulation technology such as low cost DMDs have enabled novel tissue imaging approaches. We are currently developing a portfolio of DMD-based spatial-frequency domain imaging (SFDI) platform for mapping simultaneously tissue microstructure, chromophore composition, and hemodynamics, which has vast applications in health. A real-time Single Snapshot Multiple Frequency Demodulation-Spatial Frequency Domain Imaging (SSMD-SFDI) platform has been developed and is capable of imaging and monitoring dynamic turbid medium and processes over a large field of view. When imaging forearms of healthy subjects under the reactive hyperemia protocol with this SSMD-SFDI platform, our approach not only successfully decouples light absorption by melanin from that by hemoglobin and yields accurate determination of cutaneous hemoglobin concentration and oxygen saturation, but also provides reliable estimation of the scattering properties, the melanin content and the epidermal thickness in real time[12].

Figure 3: Schematic diagram of the SSMD-SFDI imaging system.

Figure 4: (a) Oxygenated hemoglobin concentration, (b) deoxygenated hemoglobin concentration, (c) total hemoglobin concentration, and (d) blood oxygen saturation for a typical subject under the forearm reactive hyperemia protocol.

Figure 5: The recovered melanin concentration and the epidermal thickness under the forearm reactive hyperemia protocol.

The thrust for cancer screening is on high spatial frequency modulated imaging (HSFDI) for a wide-field mapping of tissue subwavelength microscopic features based on our recent breakthroughs [13, 14]. Our results find the noninvasive and label-free HSFDI can successfully image the tissue microstructure from quantifying the phase function of light scattering over a large field of view and discriminate normal, inflammatory and cancerous tissue, demonstrating a great potential for in situ and endoscopic large field cancer screening.

On-going research projects:

• DMD-based spatial-frequency domain imaging (SFDI) platform for mapping simultaneously tissue microstructure, chromophore composition, and hemodynamics (blood flow, blood oxygenation, and metabolic rate of oxygen) with applications in skin imaging, cancer margin detection, fundus imaging, and endoscopic screening
• Investigate the possibility of "tagging" diffusing light by a second wave (acoustic or thermal wave) to study the local structure and dynamics in heterogeneous materials.

Selected Publications

[1]   M. Xu, W. Cai, M. Lax, and R. R. Alfano. A photon transport forward model for imaging in turbid media. Opt. Lett., 26(14):1066-1068, 2001.

[2]   M. Xu, W. Cai, M. Lax, and R. R. Alfano. Photon migration in turbid media using a cumulant approximation to radiative transfer. Phys. Rev. E, 65:066609, 2002.

[3]   W. Cai, M. Xu, and R. R. Alfano. Analytical form of the particle distribution based on the cumulant solution of the elastic Boltzmann transport equation. Phys. Rev. E, 71:041202, 2005. (10 pages).

[4]   W. Cai, S. K. Gayen, M. Xu, M. Zevallos, M. Alrubaiee, M. Lax, and R. R. Alfano. Optical tomographic image reconstruction from ultrafast time-sliced transmission measurements. Appl. Opt., 38(19):4237-4246, 1999.

[5]   M. Xu, M. Lax, and R. R. Alfano. Time-resolved Fourier optical diffuse tomography. J. Opt. Soc. Am. A, 18(7):1535-1542, 2001.

[6]   W. Cai, M. Xu, and R. R. Alfano. Three dimensional radiative transfer tomography for turbid media. IEEE JSTQE, 9:189-198, 2003.

[7]   M. Xu, M. Alrubaiee, S. K. Gayen, and R. R. Alfano. Three-dimensional localization and optical imaging of objects in turbid media using independent component analysis. Appl. Opt., 44:1889-1897, 2005.

[8]   M. Xu, M. Alrubaiee, S. K. Gayen, and R. R. Alfano. Optical imaging of turbid media using independent component analysis: Theory and simulation. J. Biomed. Opt., 10:051705, 2005.

[9]   M. Alrubaiee, M. Xu, S. K. Gayen, and R. R. Alfano. Tomographic imaging of scattering objects in tissue-like turbid media using independent component analysis. Appl. Phys. Lett., 87:191112, 2005.

[10]   M. Xu, M. Alrubaiee, S. K. Gayen, and R. R. Alfano. Optical diffuse imaging of an ex vivo model cancerous human breast using independent component analysis. JSTQE, 14:43-49, 2008.

[11]   Binlin Wu, W. Cai, M. Alrubaiee, M. Xu, and S. K. Gayen. Time reversal optical tomography: locating targets in a highly scattering turbid medium. Opt. Express, 19:21956-21976, 2011.

[12]   Xinlin Chen, Weihao Lin, Chenge Wang, Shaoheng Chen, Jing Sheng, Bixin Zeng, and M. Xu. In vivo real-time imaging of cutaneous hemoglobin concentration, oxygen saturation, scattering properties, melanin content, and epidermal thickness with visible spatially modulated light. Biomed. Opt. Express, 8:5468-5482, 2017.

[13]   M. Xu, Zili Cao, Weihao Lin, Xinlin Chen, Longfei Zheng, and Bixin Zeng. Single snapshot multiple frequency modulated imaging of subsurface optical properties of turbid media with structured light. AIP Adv., 6(12):125208, 2016.

[14]   Min Xu. Diagnosis of the phase function of random media from light reflectance. Sci. Rep., 6:22535, 2016.